Just Intonation and W.A. Mathieu’s “Harmonic Experience” 1997
by Jeff Brent © 2013

From the "Harvard Dictionary of Music" article 'Just Intonation'

Problem #1
“It is not possible to construct a diatonic scale in which both thirds and fifths are pure.”

Problem #2
“… if chromatic pitches are to be included in the system to any significant degree so as to permit modulation and transposition of the kind familiar in tonal music […] ultimately an infinite number of pitches within the octave would be required.”


Solving the problems:

With Steinberg's Hermode technology, all chord and scale tones in any instrument may be adjusted to be in tune to just intonation.

Due to commas, a real time just intonation synthesizer would (theoretically) only be capable of being played with other synthetic instruments possessing the same tuning system and/or with traditional instruments capable of continuously variable pitch (violin family, trombone, human voice, etc).

One would presume that fixed pitch 12TET instruments would have too many clashes due to the various commas (too many cents between any two “unisons” (or “siblings” as coined by Mathieu), except for the current tonality’s root, fifth, fourth, and major second).

Two notes sounded together that are like 20 cents off are way more than merely noticeably out-of-tune.

Which would leave piano and guitar out in the cold. But it turns out that Hermode tuning is compatible with 12TET!

They figured it out. Listening to their demos is a real ear-opener.


Here is one example of how this functions in the key of C:

Playing the F chord’s root (bar 1 beat 3), with a sustained A in one of its upper voices (FA), holding said A note while moving to a new D chord root (bar 2 beat 1) causes the A to readjust to accommodate the new chord (shifting the FA 22 cents to CGDA).

Where FA = the fifth harmonic of F, and
FCGDA = the third harmonic of FCGD

FCGDA
equals
the 3rd harmonic of D
which is the 3rd harmonic of G
which is the 3rd harmonic of C
which is the 3rd harmonic of F

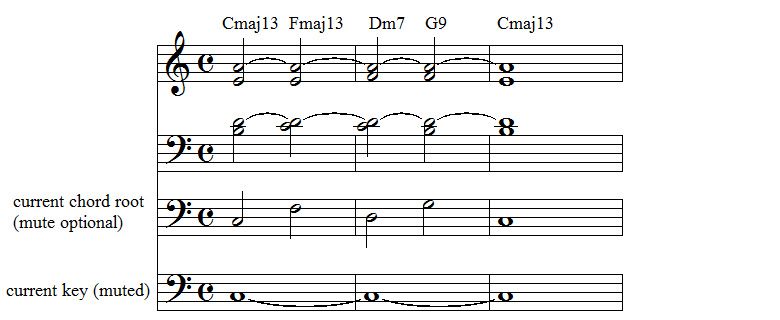
In the simple diatonic example above, the FCGD note cannot be altered to fix the wolf fifth (the “fifth” between that FCGD and the FA), because the key of C’s D is a holy grail note (9:8 – ie. common to both the pythagorean and just intonation tunings, and a barely perceptible 4 cents flat in 12TET).

Therefore, only the A can be altered to accommodate the necessary change to adjust to the new resonance requirements.

How annoying is the sudden jump of 22 cents from the FA (5th harmonic of F) to the FCGDA (third harmonic of FCGD)?

Surprisingly little. Any tension created by the shift is immediately resolved in a pleasant manner upon arrival at the next chord.

~

In the following references to W.A. Mathieu’s book “Harmonic Experience”, I am primarily only concerned with Part One, which is the section outlining his theory of Just Intonation (JI).

Harmonics Generating Harmonics
back to the Table of Contents

Just intonation, by definition, is simply a system of tuning.

The word “intonation” specifically means “a system of tuning”. The word “just” in this context means “according to the harmonic series”.

It is not, and cannot be, a mechanism which, centered at C, generates the natural heptatonic C major scale.

Throughout Mathieu’s book “Harmonic Experience” the note C is considered central with F below and G above.

The note C, however, is incapable of generating F either via the harmonic series, harmonics of harmonics, or by any other means.

The F (as described in Mathieu’s work) which is related to C is termed “reciprocal”. Used in this sense, "reciprocal” (as defined by the author) means that F is the generating tone (the generator) and that C is a generatee, ie. the tone being generated.


This evolves into much more convoluted and complex forms as Mathieu’s book advances and one begins encountering reciprocals of reciprocals and overtones of overtones (not to mention overtones of reciprocals and reciprocals of overtones).


If C is generated by F, then clearly C cannot be the ur-generator (aka ‘central note’) of the C major scale.

The “acoustic root” of the triumvirate

F – C – G

must therefore be F in the dubious context of “harmonics generating harmonics”.

(Which would suggest that perhaps Lydian ought to be regarded as the ur-scale, and not the Ionian/Major – a concept which has historically had more detractors than advocates.)


It is, in fact, true that harmonics generate harmonics. But past a certain point, those harmonics become imperceptible to all but the most sensitive precision machines (and not at all perceptible to the human ear). Indeed, harmonics were completely unknown to European scientists until the early 1700s.

The concept of harmonics generated by harmonics is simply a mathematical artifact and divorced from the physical world of human perception.


IF, however, we did accept that the ur-generator of the C major scale, is indeed F, then F generates C; and the C generated by F (FC) must then generate G (FCG).

Because C is the third harmonic of F, and G is the third harmonic of C.


Is the G (third harmonic of FC) perceptible to the human ear from playing a fundamental F?

Further, is the E (as fifth harmonic of FC) perceptible to the human ear from playing a fundamental F?

And even further, is the B (fifth harmonic of FCG) perceptible to the human ear from playing a fundamental F?

I would have to say “no” to all the above.

Because if the answer were “yes”, then by merely playing a solitary low F fundamental, the human ear would be able to discern/experience the entirety of the C major scale at one whack in all its glory.


In other words:

F begets FC (and FA)
ie. FC is the third harmonic of F (and FA is the fifth harmonic of F)

FC begets FCG (and FCE)
ie. FCG is the third harmonic of the third harmonic of F (and FCE is the fifth harmonic of the third harmonic of F)

FCG begets FCGD (and FCGB)
ie. FCGD is the third harmonic of the third harmonic of the third harmonic of F (and FCGB is the fifth harmonic of the third harmonic of the third harmonic of F)

Or seen in the illustration below (where middle C = 4C)

1F begets 3C (and 3A)

3C begets 4G (and 5E)

4G begets 6D (and 6B)

So, our fundamental 1F supposedly “generates” 6B (5 1/2 octaves above)?

C’mon! This might be mathematically possible, but in the actual physical world of the human ear there is no justification for this due to the imperceptibility (inaudibility) of those extremely low volume upper partials (especially if the “generating” fundamental is any higher than, say, 2G, where the upper partials begin to exceed the range of human hearing).

Just Intonation is not a generative system for deriving the C major scale (whether or not one accepts “F” as its acoustic root).

Harmonics generated by 5th harmonics
back to the Table of Contents

Riddle me this:
If, indeed, third harmonics (and third harmonics of third harmonics) did generate perceptible harmonics, why wouldn’t it then also be acceptable to posit that fifth harmonics can generate perceptible harmonics too?

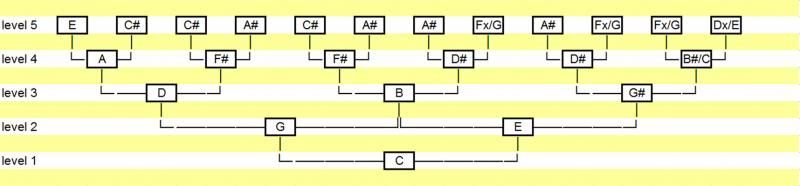
In the graphic below, you can see exactly what happens in such a hypothetical situation:

The example above proves that the harmonics derived from the fifth harmonics are worthless for describing any of the most common and useful scales and/or chords in music, except for the diminished chords and scales.

Also note especially that there is nary an F in sight.


It is interesting to notice that level 5 is a diminished 7th chord, as is level 4 (but a different one).

Combining levels 4 and 5 results in a diminished scale.

Level 3 is a diminished triad.

Combining levels 3, 4 & 5 results in an incomplete 11-note chromatic scale subset that is conspicuously lacking the F.

Combining levels 3 and 4 results in an incomplete 7-note diminished scale (no F).

Combining levels 2, 3 & 4 results in some crazy nonatonic scale: C D D# E F# G G# A B

Combining levels 2 and 3 results in an equally uncommon scalar pentatonic: D E G G# B, but whose pitch set is identical to
E7#9 - E G# B D G.

Combining levels 1, 2 & 3 results in an uncommon Cmaj9b13 chord C E Ab(/G#) B D G. Does this pitch set represent any common hexatonic scale? Nope.

Combining levels 1 and 2 results in a C major triad.


Based on this evidence, it is clear (in this context) that fifth harmonics can be (theoretically/ mathematically) generated by third harmonics, but that those fifth harmonics’ harmonics themselves don’t actually contribute anything useful vis-à-vis common musical structures other than said diminished structures (that is, if you first buy into the notion that fifth harmonics generate harmonics).

Fourth harmonics are redundant, so this yields an inherent limiting factor on the type of entities that can be considered as generators in the just intonation system of tunings.

The fifth harmonics can be generatees but not generators.

The limit is therefore the third harmonic - the highest possible harmonic that may theoretically act as a generator.



A physical illustration of just how inaudible the 5th harmonic is:

Play the C two octaves below middle C, and while sustaining that note, play the Eb immediately above middle C. If the 5th harmonic were actually audible to any significant degree, this Eb note would clash horribly with that C’s 5th harmonic E (the E immediately above middle C) – thus making the standard garden variety C minor chord impossible to listen to without cringing.

Yet we all know that minor chords are one of the staples of music. The 5th harmonic has no effect whatsoever on the beauty of minor chords.

The Sub-Dominant
back to the Table of Contents

Mathieu pg 44

“The name ‘subdominant’ has been persistently misunderstood by generations of theory students: Most musicians, even well-educated ones, think that the subdominant is so named because it is the fourth degree of its scale, just one degree below the dominant, which is its fifth degree. […] The ‘sub’ of subdominant actually refers not to its scalar position but to its harmonic reciprocity”

While it is certainly fun to think of the subdominant as being “the dominant below”, the moniker ‘subdominant’ is quite simply based on the Roman Numerals of the scale degrees: The dominant is the fifth degree of the scale (dominant = V), the Roman Numeral “IV” literally means “5 minus 1 – ie. ‘the dominant subtract one’,” which is to say the sub-dominant.

I find Mathieu to have a very active imagination, especially when it comes to items that he does not fully understand. There is more evidence of this later, for example when he rants on for a page about why the term “diminished 7th chord” is not the correct name for the structure built by stacking minor thirds (pg 372).

The Listener’s Point of View vs The Performer’s Point of View
back to the Table of Contents

I’ve spent a good deal of time in my life in lands that use non-tempered tunings (JI tunings?), I have experienced these musics first-hand up-close and personal. I've hung out with the musicians while in Morocco, Egypt, Lebanon, Syria, Greece, Turkey, Pakistan and India. For the three years I lived in the Canary Islands, I listened to music on the Moroccan radio stations morning and night.


Since I didn’t really understand the cultural structure of their musics (having only studied western theory at university at that point in my life), I didn’t attempt to try to analyze it, I simply accepted it all at face value and enjoyed it (or not) as, I must assume, any non-musician would.

I don’t ever recall saying to myself while in those countries, “Wow, those guys sound so much more in-tune than the stuff I was raised on”.


For the vocalist, Mathieu touts the boon that when one sings in perfectly pure low prime ratios that there is an accompanying wonderful sensory experience that is not achieved any other way.

If I channel “Reveille”, I more closely approximate the JI M3 than if I channel “Doe a Deer” (which nets a major third closer to 12TET or pythagorean). Though in either case, I fail to cop a buzz …

On drums, “burying the beat” (where you are so deep in the pocket that the metronome becomes completely inaudible) is a place of incredible groove that I have experienced and so I must assume that singers capable of achieving this trick must be high all the time.

Cool. More power to ‘em.


In 2011, I laid out an analysis in spreadsheet representation of Jairazbhoy’s Hindustani 32 thaat system. This gave me some intellectual insight into this facet of Indian music and the construction of their scales (thaats).

Quite coincidentally, a short time later that same year, I began working with a gentleman from Mumbai who wanted to learn his native classical Indian music on the violin. We used the Hindustani notation system (sa re ga ma pa dha ni, komal/tivra/suddha, etc), thereby increasing even more my familiarity with sargam (the Hindistani solfeggio – abbreviated from the first four notes of their equivalent of the our major scale: sa|re|ga|ma = sargam).

In addition to improvising over the various thaats in the idiom, we worked together quite a bit transcribing Hindustani violin pieces directly from recordings. Most of the ten named mainstream thaats were included in these tunes by my student’s favorite violin master (wish I could remember his name). The student stayed with me for almost a year before moving out of SoCal.

As part of my preparation for these violin lessons, I watched many many videos on the subject of Hindustani violin techniques and conventions, and the knowledge I gained in this area was fascinating.

Was my student playing pure intervals or approximative intervals on the violin? Well, since he was a student and not a professional, usually all that they are ever capable of is approximative.

Was *I* playing pure or approximative notes on the violin? Once again, probably approximative. Please note that many of the pieces I worked out in advance to prepare for the lessons, I figured out on the piano (and had no problem with any notes “falling between the cracks”).

No, I am not a classically trained Hindustani violinist, and I’m sure that a true Indian master violinist would have much to criticize regarding my approach to their music. But that is not the point here - The important thing is that both teacher and student were playing actual music that both sounded and felt like the real deal (without the “benefit” of worrying about just intonation).

Did the fact that we were not “resonating” “pure” intervals hinder our understanding and enjoyment of our endeavors? Nope.

I would also venture to assert that musicians playing extermal instruments do not achieve musical nirvana by playing just intonation intervals on their respective axes, that is to say that this “resonant experience” associated with JI appears to only exist quite literally only inside the singer’s head.

Based on my experiences all over this planet with ‘world’ music, I also remain unconvinced that this “enhanced consciousness” achieved by singing in just intonation is transferred to audience members.

Playing just intonation intervals on the violin, does not give me a rush of ecstasy.

I do, however, regularly achieve musical bliss when playing with guys who totally kick ass and we all slip into the alpha state together. But that music is all conceived in 12TET – nothing JI about it.

Ratios: Simple vs Complex
back to the Table of Contents

Pg 13

“The simpler the vibrational pattern, the more we tend to term it ‘consonant’; the more complex the pattern, the more we use the term ‘dissonant’.”

Here is a nice complex ratio: 16384/10935

Is this ratio consonant or dissonant?


The difference of only a couple of cents produces extremely complex ratios, but which are still considered VERY consonant (and NOT dissonant) because they approximate ‘ideal’ consonance so well.

702 cents (just P5) vs 700 cents (tempered P5)

3/2: 702 cents (just P5)

16384/10935 = ca. 700.0012801 cents (tempered P5)
(2 to the 14th power)/(3 to the 7th power x5)

[Thanks to Schell Barkley for the math!]


Mathieu’s statement above regarding the nature of consonance and dissonance is at the very crux of the just intonation argument, is here proven false.


A better definition of the human perception of “consonance” is in order.

“Consonance”, as perceived by humans, must obviously be a separate ‘quality’ from the perception of “resonance” (Mathieu’s term for singing pure intervals), with “resonance” being a very specific term for the intonation of small number prime ratios, and “consonance” being a broader and more flexible term.

We perceive an equal-tempered fifth as being perfectly consonant in spite of the fact that it is not 100% perfectly resonant.

As above, Mathieu states:
“The simpler the vibrational pattern, the more we tend to term it ‘consonant’; the more complex the pattern, the more we use the term ‘dissonant’.”

This boils down to that same myth:

Consonance = simple ratios
Dissonance = complex ratios

When hearing two notes played or sung by two different musicians as a “unison”, the human ear has a tolerance of about 3 cents either way before it begins to hear any degree of clashiness.

There are an infinite number of complex fractions that will approximate a tone somewhere in the range of 699 to 701 cents above a fundamental (ie. a tad flat to the 3:2 perfect fifth).


The reason scientific and music facts are called “theory” is simply because proving those facts true for all instances is generally impossible due to the infinite number of instances – yet it only requires one sole example to prove something false.

Here it's been proven that the simplicity (or complexity) of a ratio is by no means the only consideration in the human perception of consonance.


It is also commonly postulated that The human ear when presented with an interval that closely approximates one of the ‘pure’ intervals of the lower harmonic series, automatically assigns the approximative notes to those of the ‘ideal’ interval.

There are a couple of problems with this worldview as well:

- In the lower harmonic series, the 7th harmonic (while its “purity” is not in dispute) is considered by very few to be “ideal” – whether juxtaposed against the fundamental OR the 5th harmonic M3.

- Also in the lower harmonic series, there exists no half-steps, so referencing the lower harmonic series as the template for all intervals (consonant or otherwise) might not be a broad enough palette.



Observations:

1. There is a difference between pure resonance and human perceived consonance. There are those who would assert that our ancestors sang only in pure intervals.

Are we to believe that the great difficulty we modern humans have accurately singing pure resonant intervals is solely due to having listened to tempered music all our lives?

No. I believe that ancient humans were just as happy with approximative intervals as we are today – AND that they weren’t any better at hitting pure resonant intervals than we moderns are – without a whole lot of practice, that is.


2. Note that not all resonant intervals ("resonant intervals" defined by Mathieu as being somehow or another related to the keynote of the current tonality and having the simplest ratio possible) are consonant. Resonance (except in specific instances) does not, per se, equal consonance.


3. Also, as explained above, consonant intervals are not always resonant. Consonance, while always found in the neighborhood (or clump) of some resonant node or other, cannot equal resonance if approximative tones are, in fact, “good enough”.


4. Dissonant intervals can be sometimes heard as consonant, in certain contexts (eg. the 7b9 chord). Contextual environments consequently create huge gray areas in a music theorist’s ability to define almost any interval positively as either consonant or dissonant. Except for the extreme cases, consonance and dissonance are subjective relative qualities.


5. Resonance, while absolute, has many variations. For example, Ab has several resonant nodes in close proximity to one another (49:32, 14:9, 25:16, 128:81, 8:5, 13:8) all of which can be related, through whatever convoluted means (via reciprocals of reciprocals, overtones of reciprocals, reciprocals of overtones, and overtones of overtones, etc, etc) to ‘central’ C.

The 800 cent Ab also falls within this clump of nodes.

It must be noted at this point that all of these different Ab notes are perceived as (more or less) the same “quality” by the human ear.


6. It must also be observed that the tones commonly associated with the 12-tone chromatic scale tend to “clump”, ie. nodes which approximate each other tend to occur in close proximity, separated by relatively large regions of “nothingness” in between.

The significance of this “clumping” is that any node within the clump (or any tone falling within the limits of the clump) will be assigned by the ear to the perceived “umbrella” interval within that clump’s neighborhood. Which also helps to explain why approximative is “good enough” for most musical intents.

The Relationship between Ab and C
back to the Table of Contents

On pg 48, instructions are given on how to derive C as the fifth harmonic of Ab, where this Ab is identified as “reciprocal to C”.

“… in order to generate Ab [from C] , we pretended that it [Ab] was the tonic, even though the larger context is C. We are not really in Ab. That is precisely the trick of perception implicit in reciprocal harmony.” …“This is a sophisticated, deeply musical skill that most musicians in the world do quite intuitively.”

So the trick is pretending? This is a skill that is sophisticated - yet intuitive too? How sophisticated can anything be if it’s also intuitive? If it’s so intuitive, why do we have to play pretend?

And where is the statistical data to back up the outrageous assertion that most musicians do this without thinking (via intuition)?

On the very same page, the author states that “just major thirds may seem too low – or too narrow – for ears conditioned to equal temperament.”

Riddle me this:
If MOST musicians in the world sing reciprocal just major thirds intuitively, how is it that for those raised on equal temperament (MOST musicians in the world nowadays, I believe) a major third in just intonation may sound wrong? Which is it: Most musicians intuitively sing just major thirds – OR – just major thirds sound incorrect to most musicians?

And once again where is the statistical data to back up either of these contradictory claims?

All musicians in the Americas, Western Europe and much of Northern Asia are raised on ET. Is it Mathieu’s contention then that there are significantly more musicians in non-western countries than there are in the western world? Which inevitably leads to the questions “What IS a true musician vs. what is an uneducated hack”? How many ‘real’ musicians are there in the world, and how many of those are of the 12TET persuasion and what percentage are Just Intonators?

The Harmonic Major
back to the Table of Contents

Pg 51

Here Mathieu introduces the very first seven-note scale in the entire book: The Harmonic Major

In western musics, the Harmonic Major is the very least used scale of all. In Jairazbhoy’s 32 thaat system , it is also not a scale that is in common use (there isn’t even a name for this scale in Hindustani music).

I don’t understand Mathieu’s motivation for introducing as the very first seven-note scale in the book one that almost never gets used by either western OR eastern musicians. Maybe common and useful structures are not really the focus of this book.

5-limit Tuning Two Millenia Ago?
back to the Table of Contents

As mentioned earlier, harmonics were unknown to the European scientific community until 1700 (and not in the music community until decades later). On page 67, Mathieu states that (to the best of his knowledge) the first formulation of a 5-limit lattice in just intonation did not appear until as late as 1885 (attributed to Alexander Ellis).

However, right above that statement, he makes the unsubstantiated historical claim that 3-limit and 5-limit tuning systems had been employed and quantified more than two thousand years earlier. I would very much like to know by who, where and when these were written down – iow, Please don’t say anything you can’t back up.

“Ellis, among many of his generation, was chafing against the musical compromises imposed by twelve-tone equal temperament; he wanted to desperately believe that music of the common practice period (roughly the eighteenth and nineteenth centuries), although conceived in equal temperament, could be realized more beautifully and powerfully with an elaborately extended five-limit lattice. The impossibility of that [ie. realizing common practice classical music in just intonation tuning] has already been elegantly demonstrated …”

12TET did not begin to appear in European instruments until late in the 17th century. By the middle of the 18th century it had become more widespread, but England was still using meantone tuning up through the mid-1800s, and did not adopt ET until the halfway through the nineteenth century! Ellis was British and lived 1814-1890. He was present in the midst of the changeover in Britain from the old tuning system to the current one. This would certainly have colored his worldview.

It is interesting to note, that it was proven impossible (1985-Blackwood) to realize common practice classical music in just intonation, which (once again) echoes the Harvard Dictionary of Music quote at the very top of this article (Hermode Tuning notwithstanding).

Note that if not for tempered tunings, European classical music (and American jazz) could never have existed.

Ascending Melodic Minor
back to the Table of Contents

Page 81

The ascending melodic minor ”… generates gorgeous compositions in North Indian music …”. [But,] ”European classical music uses it [the ascending melodic minor] only fleetingly.”

If the ascending melodic minor is so popular in Hindustani music, then why is it not one of the named thaats?

The statement that classical music doesn’t use the ascending minor very much, just boggles the mind.

Doesn’t almost every minor piece in the entire classical repertoire from at least Bach onwards use the melodic minor? Which is to say that the ascending melodic is an extremely common fixture in a gazillion minor tunes where the melody ascends. How does that qualify as “fleeting”?

Vibrato
back to the Table of Contents

Page 90

Here Mathieu states that vibrato arises from the diaphragm.

There are two types of rapid modulations which are both used to similar effect:

1. Vibrato – pitch modulation = small wavering of pitch up or down from the main note
2. Tremolo – volume modulation = pitch remains constant, but the volume goes in and out

The diaphragm is incapable of causing pitch modulation (aka vibrato), this is the job of the larynx (aka the voice box). The diaphragm can, however, effect tremolo. This is a technique used commonly by flautists to emulate true vibrato.

If any real vocalist can explain to me how true vibrato (not tremolo) can be produced by using only the diaphragm, I’d sure like to know. But until then I maintain that it is a physical impossibility.

Calculating Just Intonation Ratios
back to the Table of Contents

Page 96


One thing I did find quite interesting was the method of calculating the JI ratios.

The perfect fifth is 3X the fundamental frequency.

The “ideal” major third is 5X the fundamental.

By multiplying or dividing by 3 and/or 5, one can relate any note one chooses to a base note – in this case C:

Up a perfect fifth [G - overtonal] is 3:1 (octave reduced = 3:2),
down a perfect fifth [F - reciprocal] is 1:3 (octave reduced = 4:3)

Up a major third [E - overtonal] is 5:1 (octave reduced = 5:4),
down a major third [Ab – reciprocal] is 1:5 (octave reduced = 8:5)

Up two perfect fifths [D - overtonal] is 3x3:1 (octave reduced = 9:8),
down two perfect fifths [Bb - reciprocal] is 1:3x3 (octave reduced = 16:9),

BUT the most ‘kosher’ Bb in just intonation is 9:5. Another Bb node (with a simpler ratio) can be found by going up two perfect fifths [overtonal] from C (3x3), and then down a major third [reciprocal] from D (1/5), which works out to 3x3:5 = 9:5.


In spite of just intonation tunings always gravitating towards the simplest ration possible for any given note, the 7:4 Bb is not considered to be the ideal b7.

And why? Because majority opinion is that it's too soft, and besides it’s in the realm of 7-limit JI (where garden variety JI uses 5-limit, ie. all ratios must only be multiplied and/or divided by either 3 or 5 - no 7s allowed).


Some quick definitions:

3-limit JI only uses up to the third harmonic perfect fifth (aka Pythagorean tuning),

5-limit JI uses both the third harmonic fifth and the fifth harmonic major third.

7-limit JI uses both the third harmonic perfect fifth, the fifth harmonic major third, and adds in the seventh harmonic b7]


As seen above, for example, to calculate one of the Bb nodes, we can (confusingly) go up two overtonal fifths and then down a reciprocal third.

Let’s take a look at how some other ratios can be found in a similar manner:

The note A can be found by going down a perfect fifth [reciprocal] from C (1:3) and then up a major third [overtonal] from F (5:1), which works out to 5:3. This is the A that resonates with F.

An A note can also be found by going up three perfect fifths [overtonal] from C (3x3x3:1) (octave reduced = 27:16). This is the A that resonates with D.

It is precisely this A’s duality which throws a monkey wrench into a diatonic scale in which both thirds and fifths are pure [à la HDM]. And precisely the reason that we humans were obliged to devise tempered scales to begin with.

Eb is up an overtonal fifth x3 and down a reciprocal major third 1/5 (or down a major third 1/5 and up a fifth x3) which is 3:5 (octave reduced = 6:5)

Db is down a reciprocal fifth 1/3 and continues down with a reciprocal major third 1/5 which is 1:15 (octave reduced = 16:15)

B is the mirror of Db. Up a fifth and up a third or up a third and up a fifth is 15/1 (octave reduced = 15:8)

In fact, all the ratios have their mirror (except one):

G = 3/1 F = 1/3
E = 5/1 Ab = 1/5
A = 5/3 Eb = 3/5
B = 15/1 Db = 1/15

Bb = 3x3/5

(Although there does exist the less popular “Bb = 1/3x3” which mirrors the holy grail “D = 3x3/1”)

This “path of least resistance” is the most direct way to both quickly calculate the simplest ratio as well as mentally visualize the relative connections back to the mother ship.

Why are there Seven Tones in a Scale?
back to the Table of Contents

Page 97

Mathieu asks “Why are there seven tones in a scale?”. His answer is “no one actually knows”.

He might not know, but there are others who do, and the answer (as it turns out) is quite simple (qv Modalogy pgs 9-10).

Mathieu vs Mozart
back to the Table of Contents

Pages 104 through 106

Here Mathieu puts forth the proposition that Mozart notated his works sloppily with misspellings.

From where I stand, his examples 15.3 (pg 104) and 15.5 (pg 105) indicate that Mozart is correctly using a D# over a C major triad as a leading tone function to the E (major third of the chord).

Mathieu insists, in no uncertain terms, that Mozart’s notating D# in this context is wrong!

Mathieu sees these passages as a C minor triad oscillating back and forth against a C major triad, and suggests (erroneously) that Mozart should have used Eb to E (instead of D# to E).

The D# does not temporarily turn the harmony into C minor – The D# is simply a chromatic embellishment to a totally unambiguous C major harmony.

If only Mozart were alive today to explain this to Mathieu …

Two Cents
back to the Table of Contents

Page 112

“… a pitch variation of one cent is virtually undetectable by human ears. A variation of two cents, however, can be heard as a change of pitch and can be felt harmonically.”

Where are the statistics to back this up? The layman certainly cannot distinguish between a 702 perfect fifth and a 700 perfect fifth. Only a very very small minority of even the most highly trained musicians have an ear sensitive enough to detect a mere two cents. Generally, one doesn’t even begin to notice any difference at all until at least 4 to 5 cents off.

Don’t believe me? Try this: Tune one guitar string to concert pitch, tune the same string on another guitar 2 cents off (sharp or flat – it doesn’t matter). Play these “unison” notes simultaneously. Do they sound out of tune with each other? No, they do not. In fact, they sound very much in-tune with each other. At most you might hear some chorus effect.

Try this too: Tune two strings on a guitar to a 700 cent fifth, and on another guitar tune a 702 fifth. Now with a blindfold on, have a friend (or an enemy – it doesn’t matter) play those two strings on one guitar and then on the other. Can you tell which is the 700 and which is the 702? Be honest here and admit that you can’t.

Even better, play both fifths on both guitars simultaneously. Do they sound out of tune with each other? No, they don’t.

The human ear’s inability to detect such small nuances of pitch works well to our advantage. If we didn’t possess this tolerance, we would never be able to listen to two vocalists attmepting to sing in “unison” without cringing. Even though virtually no one ever sings perfectly in tune, the human ear allows us enough leeway to experience the joys of music without being constantly annoyed by an over-sensitivity to pitches which, though not perfectly in tune, are very very close to each other.

Note that a plucked string, immediately after attack, will always begin a bit sharp and only starts to come into “perfect” tune as the string stabilizes. Does this physical fact cause the ear to grimace due this slight discrepancy? Not at all.

And for those of you who claim that they can hear the difference of two cents:

1. Congratulations, you’re a member of an aristocratic club with a extremely small, exclusive membership, and a better man than I,

2. I sincerely hope that your over-sensitivity to extremely small pitch nuances does not decrease your enjoyment of musicians who don’t play or sing exactly perfectly in tune (99.99% ???).

The Pentatonics, and "D" as the Central Reference Pitch
back to the Table of Contents

Pages 118-119

Throughout this book the diatonic major scale is presented as being the primordial ur-scale, with the pentatonics (A C D E G and C D E G A) being almost completely ignored. The pentatonics are basically treated as an afterthought, scales which are little more than a 7-note ionian mode with a couple of notes missing.

The ubiquitous pentatonics are given a mere three paragraph treatment out of the 500-odd pages in Mathieu's massive work. This is disconcerting given that the pentatonics are the first scales ever mentioned in any literature (239 BC) and the fact that they occur quite prominently every culture’s music all over the entire planet.

Further, the author states on page 74, that the note “D” is truly the central reference pitch of the ionian set of modes: “… the Major mode itself is not central to harmonic space; Dorian is. And D Dorian is central, both to early music and to modern nomenclature.” and “During medieval times in Europe […] the Dorian mode […] occupied the center of the harmonic aesthetic […] The note D had become, roughly, the central reference pitch. This is still true: D, not C.”

So the C major scale isn’t really central to the ionian set of modes, but the dorian is? (In spite of the fact that almost every graphic in the entire book illustrates “C” as being the center).

Most poignantly, Mathieu asserts that the note “D” is central to the entire system: The note D had become […] the central reference pitch. This is still true: D, NOT C.” [my emphasis]

BUT, there is a major contradiction between pages 74 and 118-119, if one accepts the concept of “D” being the central reference pitch (which I do, btw):

Mathieu claims on page 119, that the A minor pentatonic has a different D from C major pentatonic.

If D is the central reference pitch for the ionian set of modes (not forgetting that the 9:8 D is a holy grail note), why wouldn’t D also be the central reference pitch for the pentatonics, since the pentatonics are supposedly merely subsets of the diatonic heptatonics?

Does that mean that now there are two central reference pitches, since there are two different Ds in the pentatonics? This inconsistency is impossible for me to resolve.

Unless the common pentatonics and heptatonics are actually two different systems (the heptatonics have two different As, and the pentatonics have two different Ds). But that doesn’t make any sense either.

A ‘Sharp’ Major Third in Context preferred over a Just Intonation Major Third
back to the Table of Contents

Composer Phil Kelly once wrote "... to those NOT playing a tempered keyboard instrument, there is a minimal difference between say D# and Eb depending upon the context on the note in a given key.

i.e: in a B7 chord resolving to E , the D# will tend to "lean" a few cents to the sharp side to resolve up to the E.

However , if the same tritone is part of an F7 resolving to Bb , the Eb will consequently "lean" a few cents to the flat side to resolve DOWN to the D.

The same tendences will affect the 'natural' note regarding on the context:

in the B7 / E context, the A will lean FLAT
in the F7 / Bb context , the A will lean SHARP"

From an email exchange on this same subject, here is some insight from one of my former theory professors Paris Rutherford (Regent of Jazz Studies, University of North Texas):

Me:
I was intrigued by your statement on page 21 [of his 2008 Hal Leonard book, “The Vocal Jazz Ensemble”], regarding A’s leading tone C# being sung higher than the Db of the previous chord.

Paris:
“Competent string players understand the difference between a C# and a Db, or they will not be hired in a classy orchestra. […] a C# leading to the D or a D major chord is slightly higher pitched than the Db moving as the 7th of an Eb7 chord proceeding to the C of the Ab major chord. I tell my singers (and have for decades) treat that like a leading tone (etc., etc. whether a real leading tone or not - immaterial). After a while their ears understand and we're moving forward musically.”

I tried to find out if he meant sharp to the JI 5/4 third? Or sharp to the tempered 400 cent third? Or sharp to the pythagorean 81/64 third? But quantifying feelings into cents isn’t his musical thing.

So, in the world of classical music major thirds are noticeably sharpened.

This makes sense from the tension-resolution relationships in music – a sharper third creates greater tension and therefore a greater need to resolve. The higher the tension, the stronger the satisfaction felt when the resolution arrives.


However, there are those in the Just Intonator camp who consider the above to be debatable. That this manner of playing has more to do with perfomance practices than with the actual intonation involved.

The proof is in the pudding: Do or do not these “sharpened” thirds produce stronger movements (especially in cadences) than a “normal” third would? And why would the ear accept or much less desire this “artificial effect” in the first place, if it weren’t the natural thing to do?

The Elephant in the Room
back to the Table of Contents

Piano Stretch Tuning is the elephant in the room. It is neither just intonation, nor pythagorean, nor is it even 12TET!

Yet IT STILL WORKS GREAT!

Does the piano clash with itself so much that everyone cringes at the idea of hearing piano music? Not by a long shot. If it did, the piano wouldn’t be one of the very most popular instruments in the whole wide world, would it?

Does the piano clash with other instruments so much that people balk at the thought of listening to an ensemble that has a piano in it? Quite the contrary! The piano is historically practically ubiquituous in ensembles of all mainstream styles.

How lucky we are that the human ear is so forgiving that we can listen to all these different instruments playing simultaneously, even though the odds of perfect tuning between them are quite low, and still derive such intense pleasure from experiencing their rhythmic polyphony!

5-limit structures
back to the Table of Contents

Although many recognizable structures arise from the extensions of the 5-limit paradigm, many of those structures are kind of oddball.

We know that this array

                  F triad   C triad   G triad
5th harmonics        A         E         B

3rd harmonics        C         G         D

bases                F         C         G

contains the same exact notes as (one variation of) the C major heptatonic diatonic scale (2 redundants: C and G).

That’s cool and I like that a whole lot.

~

In this analysis our basic unit is assigned to be a triad composed of only notes which have a perfect fifth or major third relationship with a given base note.

Beginning with F as our sole base, we see that the base note generates two non-octave harmonics which are the fifth and third of a major triad.

                  F triad
5th harmonic         A

3rd harmonic         C          

base                 F          

(Zero redundants)

So, when simply playing a solo fundamental you’re actually creating a major triad (in mathematical theory at least).

That’s cool and I like that a whole lot, too.

~

Keeping F as our ur-base, add in the harmonics of its fifth degree C:

                  F triad   C triad
5th harmonics        A          E

3rd harmonics        C          G

bases                F          C

Combining the six notes of the F major triad and the C major triad yields a pentatonic structure with 1 redundant: C.

However, this pentatonic structure is not the ubiquituous common anhemitonic pentatonic that we all know and love:

C E F G A

E F G A C

F G A C E

G A C E F

A C E F G

I know of no common name nor common usage for the above five notes regarded as scalar structures.

(I really wish it had been the ur-pentatonic, that would’ve given this system magical charms.)


It does, however, translate into an Fmaj9 chord: F A C E G

But that seems a pretty sophisticated chord for cavemen to sing.

~

Here we take this structure out past the three combined major triads F, C and G and introduce the next fifth up the way:

                  F triad   C triad   G triad   D triad
5th harmonics        A         E          B        F#

3rd harmonics        C         G          D        A

bases                F         C          G        D

Combining the twelve notes of these four major triads yields an octatonic structure with 3 redundants. This pitch set is most commonly known as

G Bebop Dominant scale (mixionian) G A B C D E F F# G, or

D mixodorian (Bebop Dorian, Bebop Minor) D E F F# G A B C D

It is interesting to note that the mixodorian can also be derived by combining the major and minor pentatonics of the same root [tx db!]:

D minor pentatonic D F G A C D

D major pentatonic D E F# A B D


Adding more bases to the grid only increases both the redundancies and dense chromatic clusters.

Reciprocals and Overtonals Combined
back to the Table of Contents

If we can’t go any further out, things can only go down from here.

So far, in this section, we have only regarded combining “overtonal" triads.

Here we take a look at the properties of reciprocals, and then combining reciprocals with overtonals.


As in the previous discussion of page 96, base C is the major 17th (major third) of Ab, as well as being the 12th (perfect fifth) of F.

As seen in “the rules of engagement” above (“assigning our basic unit to be a triad composed of only notes which have a perfect fifth or major third relationship with a given base note”), our notes F and Ab still have respectively a direct perfect fifth and major third relationship with the base note C.

For this section of this analysis, our base note here is F, so F is the major 17th (major third) of Db, and F is the 12th (perfect fifth) of Bb.

              F triad

M3               A

P5               C

base             F

5P               Bb

3M               Db

              Bbm Triad

Combining the Bbm triad and the F major triad yields a 5-note stucture with "1" redundant (the base F):

Bb Db F A C

which is instantly recognizable as a BbmM9. But once again, that seems a pretty sophisticated chord for cavemen to sing.

Does that 5-note pitch set form any commonly used pentatonic scales?

Bb C Db F A

C Db F A Bb

Db F A Bb C

F A Bb C Db

A Bb C Db F

Not that I know of …

~

We had better luck with threesies. So, let’s try it this way (two overtonals with F’s reciprocal):

               F triad      C triad


M3                A            E


P5                C            G


base              F            C


5P                Bb


3M                Db


               Bbm triad

Combining the Bbm triad with the F and C major triads yields a 7-note structure with "2" redundants (base F and C).

F G A Bb C Db E F

this is the F Major Harmonic scale.

As mentioned above, the major harmonic is a very rarely used scale in any music – western or eastern.

~

Let’s try this threesie now (two overtonals with the C’s reciprocal):

              F triad       C triad


M3               A            E


P5               C            G


base             F            C


5P                            F


3M                            Ab


                            Fm triad

Combining the F major triad with the C major triad and C’s reciprocal F minor triad yields a 6-note structure with 3 redundants
(F, C and base C).

F G Ab A C E

G Ab A C E F

Ab A C E F G

A C E F G Ab

C E F G Ab A

E F G Ab A C

Do I know any fancy names for any of these hexatonic pitch collections? Nope sorry.

~

Now for a foursie, two overtonal and two reciprocal:

              F triad    C triad


M3               A          E


P5               C          G


base             F          C


5P               Bb         F


3M               Db         Ab


             Bbm triad   Fm triad

~

And the point to combining all of the above triads, in combinations of overtonals and reciprocals would be?
That, really, the very most useful musical structures created are:


1. The base with its 5th and major 3rd - which is identical to the major triad.


2. The three bases of F, C and G with their 5ths and major 3rds - which is identical to the major scale.

3. The base with its reciprocal 5th and reciprocal major 3rd - which is identical to the minor triad.


Here’s what the above permutations didn’t do:

- didn’t produce the ur-pentatonic

- didn’t produce the ascending melodic minor

- didn’t produce any diminished scales

- didn’t produce the wholetone scale

- didn’t produce the Bebop Major scale

IOW, it didn’t produce most of the scales that are common and useful in music (other than the C major scale).


Instead, it only produces:

- a maj9 chord (cool, but probably not used by the ancients)

- the Bebop Dominant scale - four overtonal stacks = G Bebop Dominant / four reciprocal stacks = Eb Bebop Dominant (also a cool
  scale, but a relatively recent addition to the vernacular)

- a mM9 (cool too, but also probably not used by the ancients)

- the harmonic major scale (rare - but it shouldn't be)

- some hexatonic scale nobody’s ever heard of (or at least I haven't)

- a hybrid harmonic minor/major scale (Did I leave something out?)

IOW, most of what else it produced was rather uncommon combinations rarely used in the history of music.

Gripes
back to the Table of Contents

1. One of my biggest critiques of Mathieu's tome is that playing through the staff notation examples requires a much higher level of pianistic proficiency than seems necessary for a book of this nature.

After doing battle with sight-reading through Mathieu’s hundreds of complicated little ‘etudes, etc’ and making the effort to get them up to minimum speed just so I could hear what they’re really supposed to sound like, I usually found myself frustrated to have gotten no real illumination from them, rather just more weird sounding ‘avant garde’ stuff - which did very little to convince me of the validity of this supposedly wonderful musicality that he so passionately promotes all throughout his work.

The grand staff examples could have been both simpler AND more straightforward, with MUCH less irrelevant and gratuituous ornamentation, and more importantly, the level of the staff examples should be geared towards the capabilities of your average non-pianist music-major’s keyboard expertise, rather than setting the technical barre so high that only a certain pianistic elite may have the benefit of being able to play at a reasonable speed without having to put in a good deal of additional practice time to bring it up to snuff.

Put simply, it’s more trouble than it’s worth. Lots of work with little or no pay-off.


2. If this book had been published only a couple of years later, it would have certainly included an audio CD with all of the staff examples played for us to listen to (along with audio of all the just intonation exercises, etc).

Mathieu wrote a 500 page book about just intonation, yet no matter what I do (whether I shake it, jump on it or throw it against the wall), I cannot HEAR a pure interval via this book. An audio CD would have made working through this book a whole lot quicker for me.

~

Below is a faithful midi rendition of the first 16 bars of the second longest composition in his book (pg 439 - 32 bars).

Mathieu "Harmonic Experience" pg 439 - first 16 bars - midi audio

~

Conclusion
back to the Table of Contents

Instead of getting bogged down in the hugely complex and confusing world that Mathieu attempts to explain in his lengthy work, Hermode Tuning does the grunt work for us via the its algorithms.

Equal Temperament was specifically invented to address the shortcomings of Just Intonation, Pythagorean, Mean Tone, and practically every other tuning system ever tried. ET works great, but music sounds even sweeter when adjusted for resonance.

And I'm all for anything that makes music sound better!

~

For those of you who have made it this far and wondered what the hubbub is really all about about, here is some bonafide audio for you so you can hear for yourself (source: wikipedia “Just Intonation”)